We make the assumption that there is more than one state of nature
and that the decision maker knows the probability with which each state of
nature will occur. Let ![]() be the probability that state j will occur.
If the decision maker makes decision
be the probability that state j will occur.
If the decision maker makes decision ![]() , her expected return
, her expected return ![]() is
is
![]()
She will make the decision ![]() that maximizes
that maximizes ![]() , namely
, namely
![]()
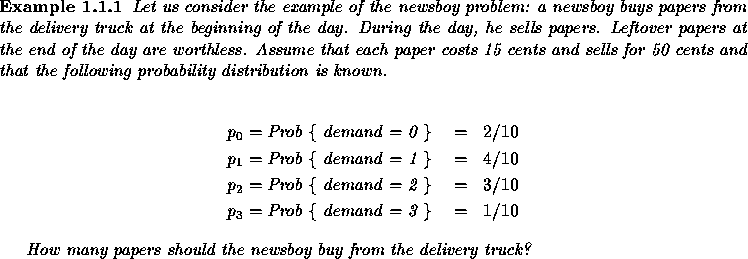
To solve this exercise, we first construct the payoff table. Here
![]() is the reward acheived when i papers are bought and a
demand j occurs.
is the reward acheived when i papers are bought and a
demand j occurs.

Next, we compute the expected returns for each possible decision:
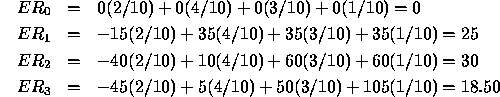
The maximum occurs when the newsboy buys 2 papers from the delivery truck. His expected return is then 30 cents.
The fact that the newsboy must make his buying decision before demand is realized has a considerable impact on his revenues. If he could first see the demand being realized each day and then buy the corresponding number of newspapers for that day, his expected return would increase by an amount known as the expected value of perfect information. Millions of dollars are spent every year on market research projects, geological tests etc, to determine what state of nature will occur in a wide variety of applications. The expected value of perfect information indicates the expected gain from any such endeavor and thus places an upper bound on the amount that should be spent in gathering information.
Let us compute the expected value of perfect information EVPI for the above newsboy example. If demand were known before the buying decision is made, the newsboy's expected return would be
![]()
![]()
It should be pointed out that the criterion of maximizing expected
return can sometimes produce unacceptable results. This is because
it ignores downside risk. Most people are risk averse, which means
they would feel that the loss of x dollars is more painful than the
benefit obtained from the gain of the same amount. Decision theory
deals with this problem by introducing a function that measures the
``attractiveness'' of money. This function is called the utility
function. You are referred to 45-749 (managerial economics) for more
on this notion. Instead of working with a payoff table containing the
dollar amounts ![]() , one would instead work with a payoff table
containing the utilities, say
, one would instead work with a payoff table
containing the utilities, say ![]() . The optimal decision
. The optimal decision ![]() is that which maximizes the expected utility
is that which maximizes the expected utility
![]()
over all i.