When there are more than two players, fundamental differences
arise. For example, the theorem about 2-person zero-sum games
which says that all equilibrium solutions have the same
payoff cannot be generalized to three players.
Once there are more than two players, a zero-sum game can have
equilibrium solutions that do not have the same payoffs. Here is an example
with three players: each player has two strategies, say A and B.
Whatever Players 1
and 2 play, the payoff vector is 
when Player 3 chooses Strategy A and it is 
when Player 3 chooses Strategy B. We will call the player that receives
the payoff of 1 the winner and the players that receive a
payoff of 
, the loosers.
In this game, although Player 3 can never be the winner, he determines
which of Players 1 or 2 will be the winner. When this situation occurs,
we say that Player 3 is a spoiler. For example, take the 1992
presidential election: 1=Bush, 2=Clinton, 3=Perot. Perot could not
win, but he could play the spoiler in this campaign. In a two-way race,
noone can play a spoiler; in a three-way race, there is room for a
spoiler.
We can represent the payoffs in a table as follows.
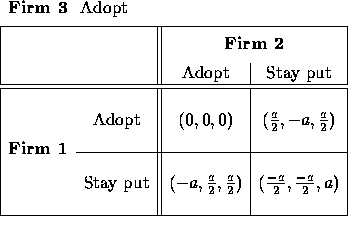
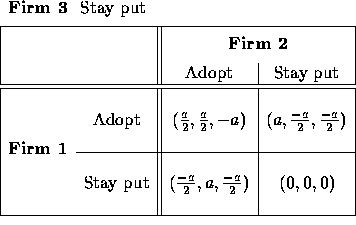
Each firm has a dominant strategy, which is to adopt the new technology. So the unique equilibrium occurs when all three firms play the pure strategy: Adopt the new technology. This is presicely what happened with two firms. No firm can be left behind in the race to adopt the new technology. This is just as true for n players as it is for two or three. So, here, the insight from the 2-person game version of Competitive Advantage generalizes nicely to n-person games.
An important aspect of n-person games is that subsets of players can form coalitions. A useful concept in this case is the core of the game. Another important aspect of game theory is that of fairness: the Shapley value provides an answer. Game theory also considers games with a sequential structure, games with imperfect information, market games (you learned about Cournot and Bertrand competition in 45-749). To learn more about these and other aspects of game theory, you are referred to the excellent book ``Games for Business and Economics'' by Roy Gardner (1995), which was used as a basis for this chapter.
